Magnitudes have long been linked to earthquakes, so it was much to everyone's surprise when magnitudes begun being linked to stars as well!
...Actually both earthquake intensities and stellar brightnesses have long been measured in magnitudes. Magnitudes are a great ways to take seemingly large, complicated, and convoluted data and convert it to something that a layperson can understand. In the case of earthquakes, the Richter magnitude scale converts seismological readings into a simple one or two digit number that clearly conveys the severity of the earthquake. Because high levels of precision aren't particularly important when discussing the intensity of an earthquake, the Richter magnitude scale employs logarithms to truncate all of the excess information.
...Actually both earthquake intensities and stellar brightnesses have long been measured in magnitudes. Magnitudes are a great ways to take seemingly large, complicated, and convoluted data and convert it to something that a layperson can understand. In the case of earthquakes, the Richter magnitude scale converts seismological readings into a simple one or two digit number that clearly conveys the severity of the earthquake. Because high levels of precision aren't particularly important when discussing the intensity of an earthquake, the Richter magnitude scale employs logarithms to truncate all of the excess information.
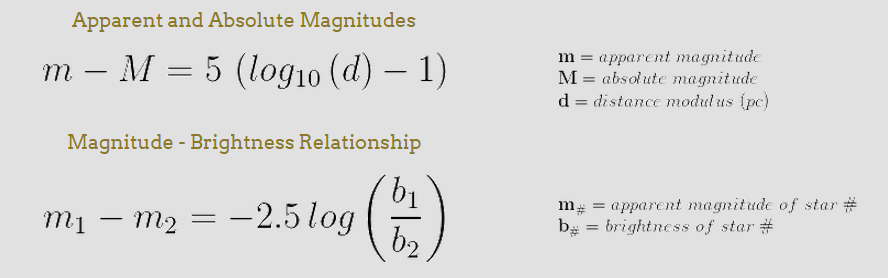
Stellar brightness is also conveniently measured in magnitudes. These magnitudes are much friendlier than the long luminosity values that we would otherwise use. For example, the sun has a luminosity of 384,600,000,000,000,000,000,000,000 Watts and an apparent magnitude of -26.7. Now, which value would you rather remember and use?
There are two types of magnitudes that you should be aware of:
Apparent magnitude: the magnitude of the object measured from Earth.
Absolute magnitude: the magnitude of the object measured at a distance of 10 parsecs away from it.
Magnitudes are not exclusive to stars, and instead can be extended to anything from Mercury to the Andromeda Galaxy.
A brief historical note on magnitudes: they have existed long before the Richter scale. Magnitudes originated early on during the Greek and Roman Empires. They were used by everyone from Hipparchus to Ptolemy, but were only standardized in the mid-19th century. The reason that the magnitude scale seems arbitrarily centered was because it involves a lot of historical artifacts.
But let's stop dwelling on the past. How does one actually calculate the magnitude of a star? As it turns out, it's quite simple! A stars apparent magnitude can be computed in one of two ways:
There are two types of magnitudes that you should be aware of:
Apparent magnitude: the magnitude of the object measured from Earth.
Absolute magnitude: the magnitude of the object measured at a distance of 10 parsecs away from it.
Magnitudes are not exclusive to stars, and instead can be extended to anything from Mercury to the Andromeda Galaxy.
A brief historical note on magnitudes: they have existed long before the Richter scale. Magnitudes originated early on during the Greek and Roman Empires. They were used by everyone from Hipparchus to Ptolemy, but were only standardized in the mid-19th century. The reason that the magnitude scale seems arbitrarily centered was because it involves a lot of historical artifacts.
But let's stop dwelling on the past. How does one actually calculate the magnitude of a star? As it turns out, it's quite simple! A stars apparent magnitude can be computed in one of two ways:







 RSS Feed
RSS Feed